HP OpenVMS Systems Documentation |
OpenVMS VAX RTL Mathematics (MTH$) Manual
Part 3
The Vector MTH$ Reference Section provides detailed descriptions of two
sets of vector routines provided by the OpenVMS RTL Mathematics (MTH$)
Facility, BLAS Level 1 and FOLR. The BLAS Level 1 are the Basic Linear
Algebraic Subroutines designed by Lawson, Hanson, Kincaid, and Krogh
(1978). The FOLR (First Order Linear Recurrence) routines provide a
vectorized algorithm for the linear recurrence relation.
|
| OpenVMS usage: | longword_signed |
| type: | longword integer (signed) |
| access: | write only |
| mechanism: | by value |
For the real versions of this routine, the function value is the index of the first occurrence of a vector element having the maximum absolute value, as follows:

For the complex versions of this routine, the function value is the index of the first occurrence of a vector element having the largest sum of the absolute values of the real and imaginary parts of the vector elements, as follows:

n
OpenVMS usage: longword_signed type: longword integer (signed) access: read only mechanism: by reference
Number of elements in vector x . The n argument is the address of a signed longword integer containing the number of elements. If you specify a negative value or 0 for n, 0 is returned.x
OpenVMS usage: floating_point or complex_number type: F_floating, D_floating, G_floating real or F_floating, D_floating, G_floating complex access: read only mechanism: by reference, array reference
Array containing the elements to be accessed. All elements of array x are accessed only if the increment argument of x, called incx, is 1. The x argument is the address of a floating-point or floating-point complex number that is this array. This argument is an array of length at least:

where:
| n | = | number of vector elements specified in n |
| incx | = | increment argument for the array x specified in incx |
Specify the data type as follows:
| Routine | Data Type for x |
|---|---|
| BLAS1$VISAMAX | F-floating real |
| BLAS1$VIDAMAX | D-floating real |
| BLAS1$VIGAMAX | G-floating real |
| BLAS1$VICAMAX | F-floating complex |
| BLAS1$VIZAMAX | D-floating complex |
| BLAS1$VIWAMAX | G-floating complex |
If n is less than or equal to 0, then imax is 0.
| OpenVMS usage: | longword_signed |
| type: | longword integer (signed) |
| access: | read only |
| mechanism: | by reference |

where:
| x | = | array specified in x |
| i | = | element of the vector x |
| incx | = | increment argument for the array x specified in incx |
If you specify a negative value for incx, it is interpreted as the absolute value of incx.
BLAS1$VISAMAX, BLAS1$VIDAMAX, and BLAS1$VIGAMAX find the index, i, of the first occurrence of a vector element having the maximum absolute value. BLAS1$VICAMAX, BLAS1$VIZAMAX, and BLAS1$VIWAMAX find the index, i, of the first occurrence of a vector element having the largest sum of the absolute values of the real and imaginary parts of the vector elements.Vector x contains n elements that are accessed from array x by stepping incx elements at a time. The vector x is a real or complex single-precision or double-precision (D and G) n-element vector. The vector can be a row or a column of a matrix. Both forward and backward indexing are permitted.
BLAS1$VISAMAX, BLAS1$VIDAMAX, and BLAS1$VIGAMAX determine the smallest integer i of the n-element vector x such that:

BLAS1$VICAMAX, BLAS1$VIZAMAX, and BLAS1$VIWAMAX determine the smallest integer i of the n-element vector x such that:

You can use the BLAS1$VIxAMAX routines to obtain the pivots in Gaussian elimination.
The public-domain BLAS Level 1 IxAMAX routines require a positive value for incx. The Run-Time Library BLAS Level 1 routines interpret a negative value for incx as the absolute value of incx.
The algorithm does not provide a special case for incx = 0. Therefore, specifying 0 for incx has the effect of setting imax equal to 1 using vector operations.
C
C To obtain the index of the element with the maximum
C absolute value.
C
INTEGER IMAX,N,INCX
REAL X(40)
INCX = 2
N = 20
IMAX = BLAS1$VISAMAX(N,X,INCX)
|
The Obtain the Sum of the Absolute Values of the Elements of a Vector routine determines the sum of the absolute values of the elements of the n-element vector x .
BLAS1$VSASUM n ,x ,incxBLAS1$VDASUM n ,x ,incx
BLAS1$VGASUM n ,x ,incx
BLAS1$VSCASUM n ,x ,incx
BLAS1$VDZASUM n ,x ,incx
BLAS1$VGWASUM n ,x ,incx
Use BLAS1$VSASUM for single-precision real operations.
Use BLAS1$VDASUM for double-precision real (D-floating) operations.
Use BLAS1$VGASUM for double-precision real (G-floating) operations. Use BLAS1$VSCASUM for single-precision complex operations.
Use BLAS1$VDZASUM for double-precision complex (D-floating) operations.
Use BLAS1$VGWASUM for double-precision complex (G-floating) operations.
OpenVMS usage: floating_point type: F_floating, D_floating, or G_floating real access: write only mechanism: by value
The function value, called sum, is the sum of the absolute values of the elements of the vector x . The data type of the function value is a real number; for the BLAS1$VSCASUM, BLAS1$VDZASUM, and BLAS1$VGWASUM routines, the data type of the function value is the real data type corresponding to the complex argument data type.
n
OpenVMS usage: longword_signed type: longword integer (signed) access: read only mechanism: by reference
Number of elements in vector x to be added. The n argument is the address of a signed longword integer containing the number of elements.x
OpenVMS usage: floating_point or complex_number type: F_floating, D_floating, G_floating real or F_floating, D_floating, G_floating complex access: read only mechanism: by reference, array reference
Array containing the elements to be accessed. All elements of array x are accessed only if the increment argument of x, called incx, is 1. The x argument is the address of a floating-point or floating-point complex number that is this array. This argument is an array of length at least:

where:
| n | = | number of vector elements specified in n |
| incx | = | increment argument for the array x specified in incx |
Specify the data type as follows:
| Routine | Data Type for x |
|---|---|
| BLAS1$VSASUM | F-floating real |
| BLAS1$VDASUM | D-floating real |
| BLAS1$VGASUM | G-floating real |
| BLAS1$VSCASUM | F-floating complex |
| BLAS1$VDZASUM | D-floating complex |
| BLAS1$VGWASUM | G-floating complex |
If n is less than or equal to 0, then sum is 0.0.
| OpenVMS usage: | longword_signed |
| type: | longword integer (signed) |
| access: | read only |
| mechanism: | by reference |
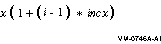
where:
| x | = | array specified in x |
| i | = | element of the vector x |
| incx | = | increment argument for the array x specified in incx |
If you specify a negative value for incx, it is interpreted as the absolute value of incx.
BLAS1$VSASUM, BLAS1$VDASUM, and BLAS1$VGASUM obtain the sum of the absolute values of the elements of the n-element vector x . BLAS1$VSCASUM, BLAS1$VDZASUM, and BLAS1$VGWASUM obtain the sum of the absolute values of the real and imaginary parts of the elements of the n-element vector x .Vector x contains n elements that are accessed from array x by stepping incx elements at a time. The vector x is a real or complex single-precision or double-precision (D and G) n-element vector. The vector can be a row or a column of a matrix. Both forward and backward indexing are permitted.
BLAS1$VSASUM, BLAS1$VDASUM, and BLAS1$VGASUM compute the sum of the absolute values of the elements of x , which is expressed as follows:

BLAS1$VSCASUM, BLAS1$VDZASUM, and BLAS1$VGWASUM compute the sum of the absolute values of the real and imaginary parts of the elements of x , which is expressed as follows:

The public-domain BLAS Level 1 xASUM routines require a positive value for incx. The Run-Time Library BLAS Level 1 routines interpret a negative value for incx as the absolute value of incx.
The algorithm does not provide a special case for incx = 0. Therefore, specifying 0 for incx has the effect of computing n*|x[1]| using vector operations.
Rounding in the summation occurs in a different order than in a sequential evaluation of the sum, so the final result may differ from the result of a sequential evaluation.
C
C To obtain the sum of the absolute values of the
C elements of vector x:
C
INTEGER N,INCX
REAL X(20),SUM
INCX = 1
N = 20
SUM = BLAS1$VSASUM(N,X,INCX)
|
The Multiply a Vector by a Scalar and Add a Vector routine computes ax + y , where a is a scalar number and x and y are n-element vectors.
BLAS1$VSAXPY n ,a ,x ,incx ,y ,incyBLAS1$VDAXPY n ,a ,x ,incx ,y ,incy
BLAS1$VGAXPY n ,a ,x ,incx ,y ,incy
BLAS1$VCAXPY n ,a ,x ,incx ,y ,incy
BLAS1$VZAXPY n ,a ,x ,incx ,y ,incy
BLAS1$VWAXPY n ,a ,x ,incx ,y ,incy
Use BLAS1$VSAXPY for single-precision real operations.
Use BLAS1$VDAXPY for double-precision real (D-floating) operations.
Use BLAS1$VGAXPY for double-precision real (G-floating) operations. Use BLAS1$VCAXPY for single-precision complex operations.
Use BLAS1$VZAXPY for double-precision complex (D-floating) operations.
Use BLAS1$VWAXPY for double-precision complex (G-floating) operations.
None.
n
OpenVMS usage: longword_signed type: longword integer (signed) access: read only mechanism: by reference
Number of elements in vectors x and y . The n argument is the address of a signed longword integer containing the number of elements. If n is less than or equal to 0, then y is unchanged.a
OpenVMS usage: floating_point or complex_number type: F_floating, D_floating, G_floating real or F_floating, D_floating, G_floating complex access: read only mechanism: by reference, array reference
Scalar multiplier for the array x. The a argument is the address of a floating-point or floating-point complex number that is this multiplier. If a equals 0, then y is unchanged. If a shares a memory location with any element of the vector y , results are unpredictable. Specify the same data type for arguments a, x, and y.
x
OpenVMS usage: floating_point or complex_number type: F_floating, D_floating, G_floating real or F_floating, D_floating, G_floating complex access: read only mechanism: by reference, array reference
Array containing the elements to be accessed. All elements of array x are accessed only if the increment argument of x, called incx, is 1. The x argument is the address of a floating-point or floating-point complex number that is this array. The length of this array is at least:

where:
| n | = | number of vector elements specified in n |
| incx | = | increment argument for the array x specified in incx |
Specify the data type as follows:
| Routine | Data Type for x |
|---|---|
| BLAS1$VSAXPY | F-floating real |
| BLAS1$VDAXPY | D-floating real |
| BLAS1$VGAXPY | G-floating real |
| BLAS1$VCAXPY | F-floating complex |
| BLAS1$VZAXPY | D-floating complex |
| BLAS1$VWAXPY | G-floating complex |
If any element of x shares a memory location with an element of y , the results are unpredictable.
| OpenVMS usage: | longword_signed |
| type: | longword integer (signed) |
| access: | read only |
| mechanism: | by reference |

where:
| x | = | array specified in x |
| i | = | element of the vector x |
| incx | = | increment argument for the array x specified in incx |
If incx is less than 0, then x is referenced backward in array x; that is, x[i] is referenced in:

where:
| x | = | array specified in x |
| n | = | number of vector elements specified in n |
| i | = | element of the vector x |
| incx | = | increment argument for the array x specified in incx |
| OpenVMS usage: | floating_point or complex_number |
| type: | F_floating, D_floating, G_floating real or F_floating, D_floating, G_floating complex |
| access: | modify |
| mechanism: | by reference, array reference |
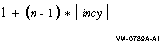
where:
| n | = | number of vector elements specified in n |
| incy | = | increment argument for the array y specified in incy |
Specify the data type as follows:
| Routine | Data Type for y |
|---|---|
| BLAS1$VSAXPY | F-floating real |
| BLAS1$VDAXPY | D-floating real |
| BLAS1$VGAXPY | G-floating real |
| BLAS1$VCAXPY | F-floating complex |
| BLAS1$VZAXPY | D-floating complex |
| BLAS1$VWAXPY | G-floating complex |
If n is less than or equal to 0, then y is unchanged. If any element of x shares a memory location with an element of y , the results are unpredictable.
On exit, y contains an array of length at least:
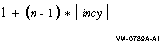
where:
| n | = | number of vector elements specified in n |
| incy | = | increment argument for the array y specified in incy |
After the call to BLAS1$VxAXPY, y[i] is set equal to:

where:
| y | = | the vector y |
| i | = | element of the vector x or y |
| a | = | scalar multiplier for the vector x specified in a |
| x | = | the vector x |
| OpenVMS usage: | longword_signed |
| type: | longword integer (signed) |
| access: | read only |
| mechanism: | by reference |
| Previous | Next | Contents | Index |